Fascinating Fractals
the intricate geometric patterns that repeat at every scale
published : 13 March 2024
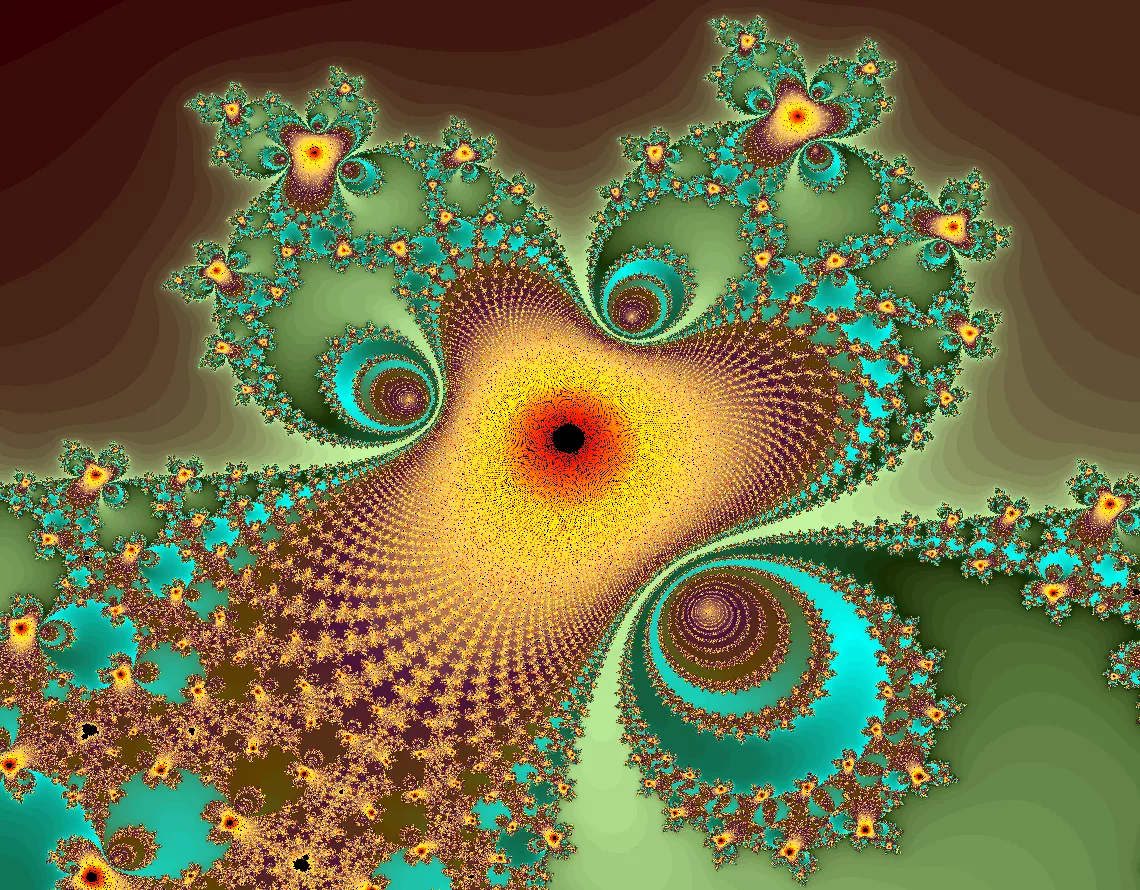
Fractals, the intricate geometric patterns that repeat at every scale, have captured the imagination of mathematicians, artists, and scientists alike. From the branching patterns of trees to the intricate designs of snowflakes, fractals are ubiquitous in nature, displaying a mesmerizing beauty and complexity.
Exploring Self-Similarity
At the heart of fractals lies the concept of self-similarity, where a pattern repeats itself at increasingly smaller scales. This property allows fractals to exhibit intricate structures that are both detailed and infinitely complex.
One of the most famous examples of a fractal is the Mandelbrot set, discovered by mathematician Benoit Mandelbrot in the 1970s. The Mandelbrot set is generated by iterating a simple mathematical formula and plotting the results on a complex plane. What emerges is a stunning array of shapes and structures, with intricate detail at every level of magnification.
The Beauty of Chaos
Fractals are often associated with chaos theory, the study of nonlinear dynamical systems. While chaotic systems are inherently unpredictable, fractals provide a way to visualize and understand the underlying order within chaos.
Chaotic systems exhibit sensitive dependence on initial conditions, commonly referred to as the butterfly effect. Small changes in the initial conditions can lead to drastically different outcomes, making long-term predictions difficult.
Fractals offer a glimpse into the underlying patterns of chaos, revealing order amidst randomness. They provide a visual representation of complex systems, allowing researchers to study and analyze chaotic behavior in a tangible way.
Applications Across Disciplines
Fractals have applications across diverse fields, from computer graphics and art to physics and biology. In computer graphics, fractals are used to create realistic textures, terrain, and landscapes. Artists use fractals as a source of inspiration, creating mesmerizing artworks that evoke the beauty of natural patterns.
In physics, fractals are used to model complex systems such as turbulence, fluid flow, and the distribution of galaxies. In biology, fractals are found in the branching patterns of blood vessels, the structure of lungs, and the growth of plants.
Unraveling Nature's Mysteries
Studying fractals offers a unique perspective on the natural world, revealing underlying patterns and structures that are often hidden from view. By analyzing fractal geometry, researchers can gain insights into the processes shaping our universe, from the formation of galaxies to the structure of DNA.
Fractals represent a bridge between art and science, offering a visual language to explore the mysteries of nature. Whether admiring the intricate beauty of a snowflake or marveling at the complexity of a coastline, fractals remind us of the inherent order and complexity of the world around us.