Function Expression :
\[f(x)=\frac{\sqrt{x+2}}{\sqrt{5x+2}} \]Domain
\[\left]- \frac{2}{5}, \infty\right[ \]Limits
\[\lim_{x \overset{>}{\rightarrow- \frac{2}{5}} }f(x) = +\infty \]\[\lim_{x \rightarrow+\infty}f(x) = \frac{\sqrt{5}}{5} \]
\[ \]
Derivate
\[f^{\,\prime}(x)=- \frac{5 \sqrt{x + 2}}{2 \left(5 x + 2\right)^{\frac{3}{2}}} + \frac{1}{2 \sqrt{x + 2} \sqrt{5 x + 2}} \]\[f^{\,\prime}(x)=- \frac{4}{\sqrt{x + 2} \left(5 x + 2\right)^{\frac{3}{2}}} \]
\[ \]
Integral
\[F(x) = \begin{cases} \frac{\left(x + 2\right)^{\frac{3}{2}}}{\sqrt{5 x + 2}} - \frac{8 \sqrt{x + 2}}{5 \sqrt{5 x + 2}} + \frac{8 \sqrt{5} \operatorname{acosh}{\left(\frac{\sqrt{10} \sqrt{x + 2}}{4} \right)}}{25} & \text{for}\: \left|{x + 2}\right| > \frac{8}{5} \\- \frac{8 \sqrt{5} i \operatorname{asin}{\left(\frac{\sqrt{10} \sqrt{x + 2}}{4} \right)}}{25} - \frac{i \left(x + 2\right)^{\frac{3}{2}}}{\sqrt{- 5 x - 2}} + \frac{8 i \sqrt{x + 2}}{5 \sqrt{- 5 x - 2}} & \text{otherwise} \end{cases} \]Sign Table
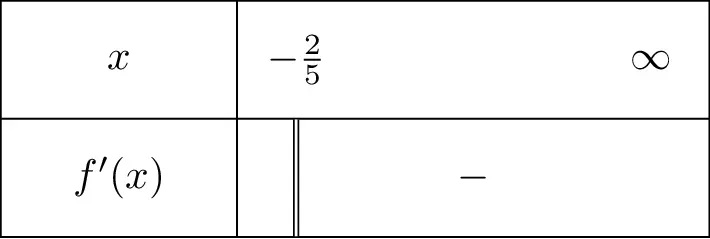
Variation Table
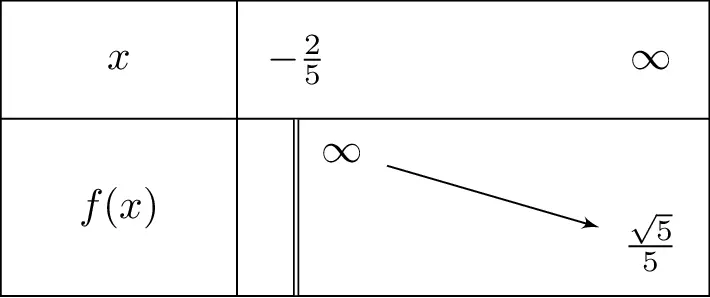
Plot
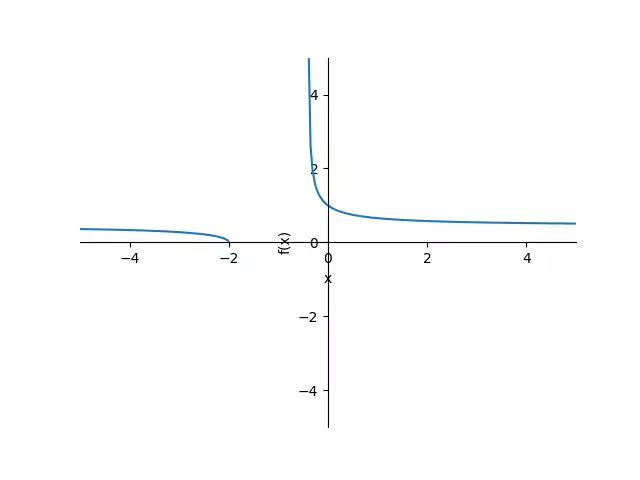
Elapsed Time: 0.0023 seconds