Function Expression :
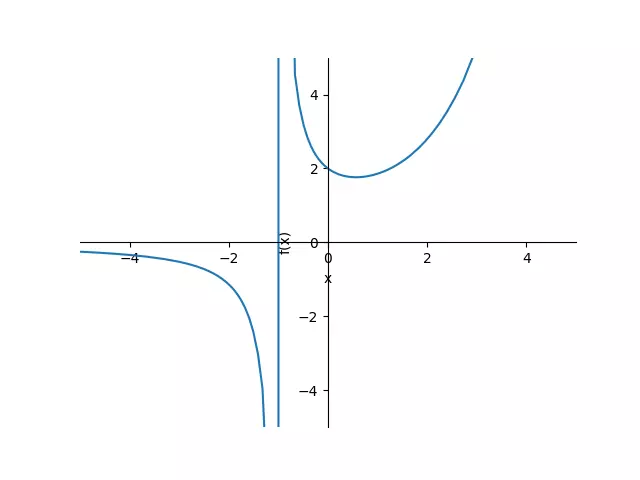
\[f(x)=\frac{e^x+1}{x+1} \]Domain
\[\left]-\infty, -1\right[ \cup \left]-1, \infty\right[ \]Limits
\[\lim_{x \rightarrow-\infty}f(x) = 0 \]\[\lim_{x \overset{<}{\rightarrow-1} }f(x) = -\infty \]
\[\lim_{x \overset{>}{\rightarrow-1} }f(x) = +\infty \]
\[\lim_{x \rightarrow+\infty}f(x) = +\infty \]
\[ \]
Derivate
\[f^{\,\prime}(x)=\frac{e^{x}}{x + 1} - \frac{e^{x} + 1}{\left(x + 1\right)^{2}} \]\[f^{\,\prime}(x)=\frac{x e^{x} - 1}{x^{2} + 2 x + 1} \]
\[ \]
Integral
\[F(x) = \int \frac{e^{x} + 1}{x + 1}\, dx \]Sign Table

Variation Table

Plot
Elapsed Time: 0.0287 seconds