Function Expression :
\[f(x)=-3xe^{2x} \]Domain
\[]-\infty ;+\infty [ \]Limits
\[\lim_{x \rightarrow-\infty}f(x) = 0 \]\[\lim_{x \rightarrow+\infty}f(x) = -\infty \]
\[ \]
Derivate
\[f^{\,\prime}(x)=- 6 x e^{2 x} - 3 e^{2 x} \]\[f^{\,\prime}(x)=\left(- 6 x - 3\right) e^{2 x} \]
\[f^{\,\prime}(x)=3 \left(- 2 x - 1\right) e^{2 x} \]
Integral
\[F(x) = \frac{\left(3 - 6 x\right) e^{2 x}}{4} \]Sign Table
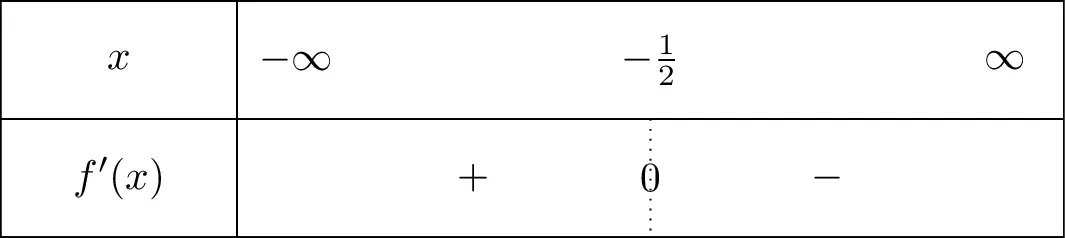
Variation Table
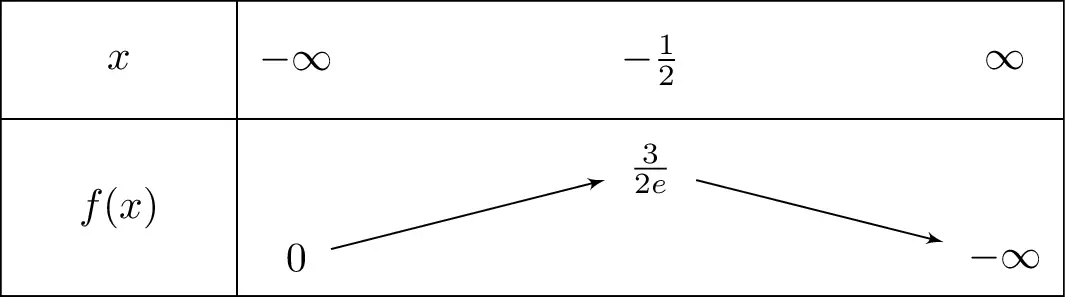
Plot
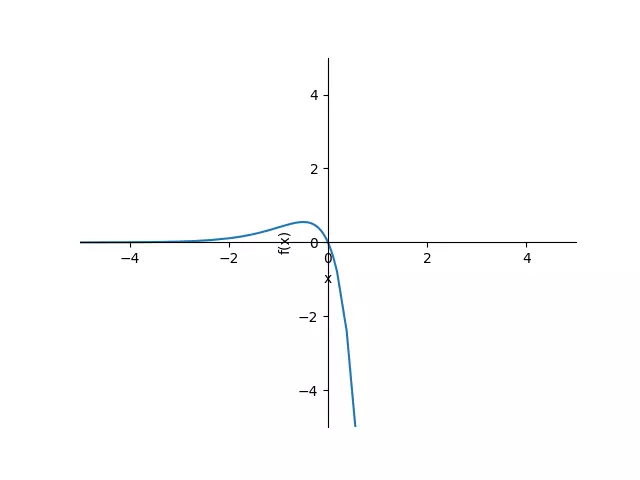
Elapsed Time: 0.0509 seconds